ChEn 433

Class 25 Hydro, Geothermal

Hydropower

  • Hydroelectric
    • 1870 Cragside Dam in Rothbury, England
    • Dams: regulating flows, flood control, storage
    • Historically used for mechanical energy
    • Renewable
    • No fuel costs
  • Wave
  • Tidal

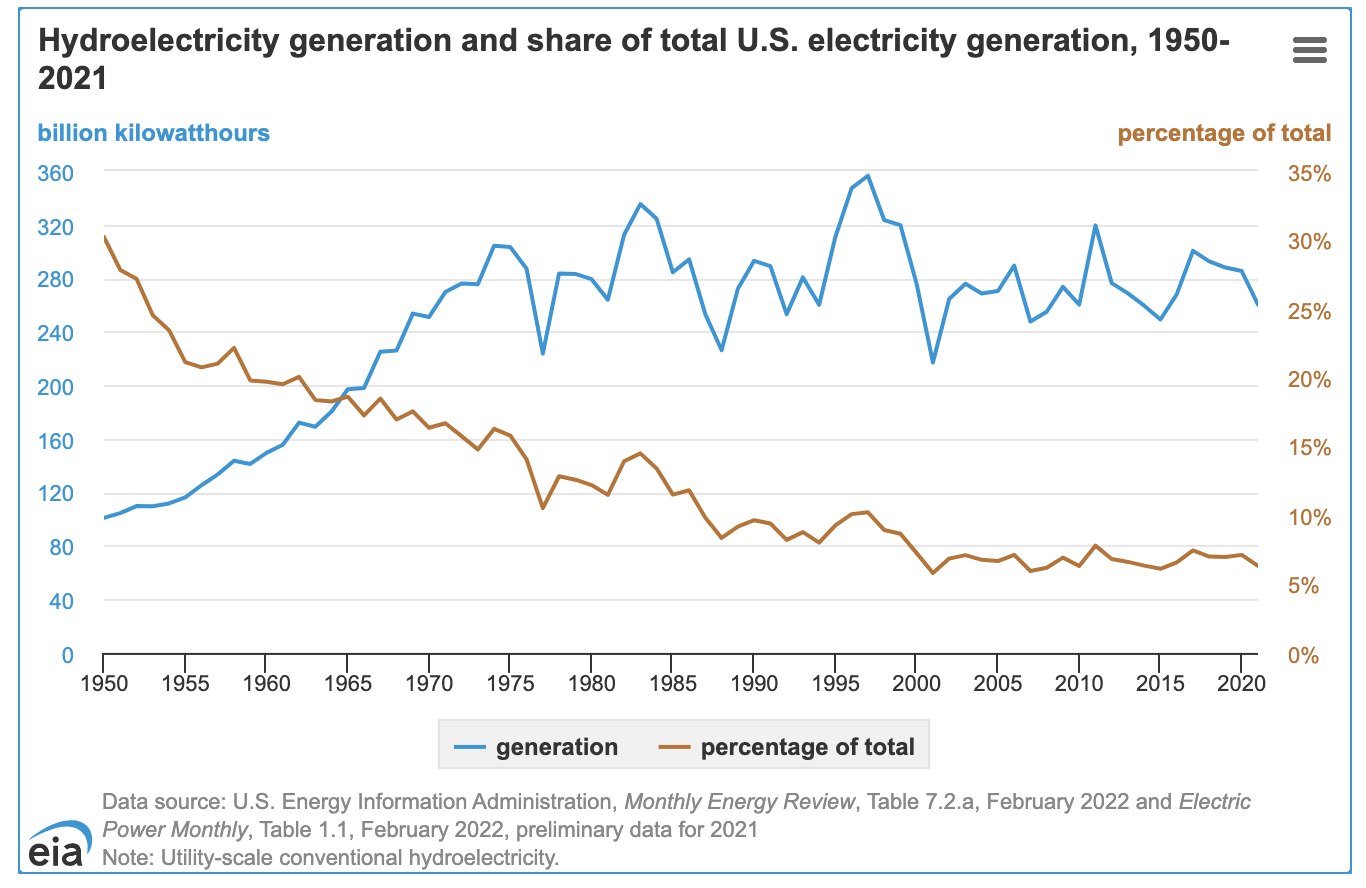
Hydropower

Hydropower

US Hydropower

US Hydropower

Three Gorges Dam

  • China
  • $32 billion
  • 181 m
  • 22.5 GW (11x Hoover)
  • Nine year construction to 2012
  • 34 Francis turbines
  • > 1.3 million people displaced
    • 1500 cities/towns/villiages along the river
  • Issues:
    • erosion, sedimentation, landslides,
    • waste,
    • wildlife

Simple Theory

\[\dot{W} = \dot{m}gh\] Power is the rate of potential energy change as the liquid falls under gravity over height \(h\).

Mechanical Energy Balance \[\dot{m}\left(\frac{P_1}{\rho} + \frac{v_1^2}{2} + gz_1\right) - \dot{W}_\text{turbine} = \dot{m}\left(\frac{P_2}{\rho} + \frac{v_2^2}{2} + gz_2\right)\]

Exercise

The average American home uses 893 kWh per month, which is 1.22 kW.

How many gallons per minute is needed to power the average home for a reservoir height of 100 m?

import pint; u = pint.UnitRegistry()

W   = 893  * u.kW*u.hr/u.month
h   = 100  * u.m
rho = 1000 * u.kg/u.m**3
g   = 9.81 * u.m/u.s**2

print(f"W = {W.to(u.kW):.2f}")

# W = mdot * g * h
mdot = W / g / h
Vdot = mdot / rho

print(f"Vdot = {Vdot.to(u.gal/u.min):.2f}")

19.75 gal/min

The average shower takes 2 gal/min

Capacity factors

The amount of power generated each year from the nation’s hydroelectric facilities varies by the water available in dams and rivers. Many reservoirs must balance power output with competing water demand for irrigation, municipal, industrial, and other needs, as well as concerns with fish migration. As a result, hydroelectric facilities often do not run at full output. U.S. hydroelectric capacity factors, which measure actual output as a percent of total capacity, average between 30% and 40%. –EIA.gov

Article: Drought

Turbine types

Turbine types

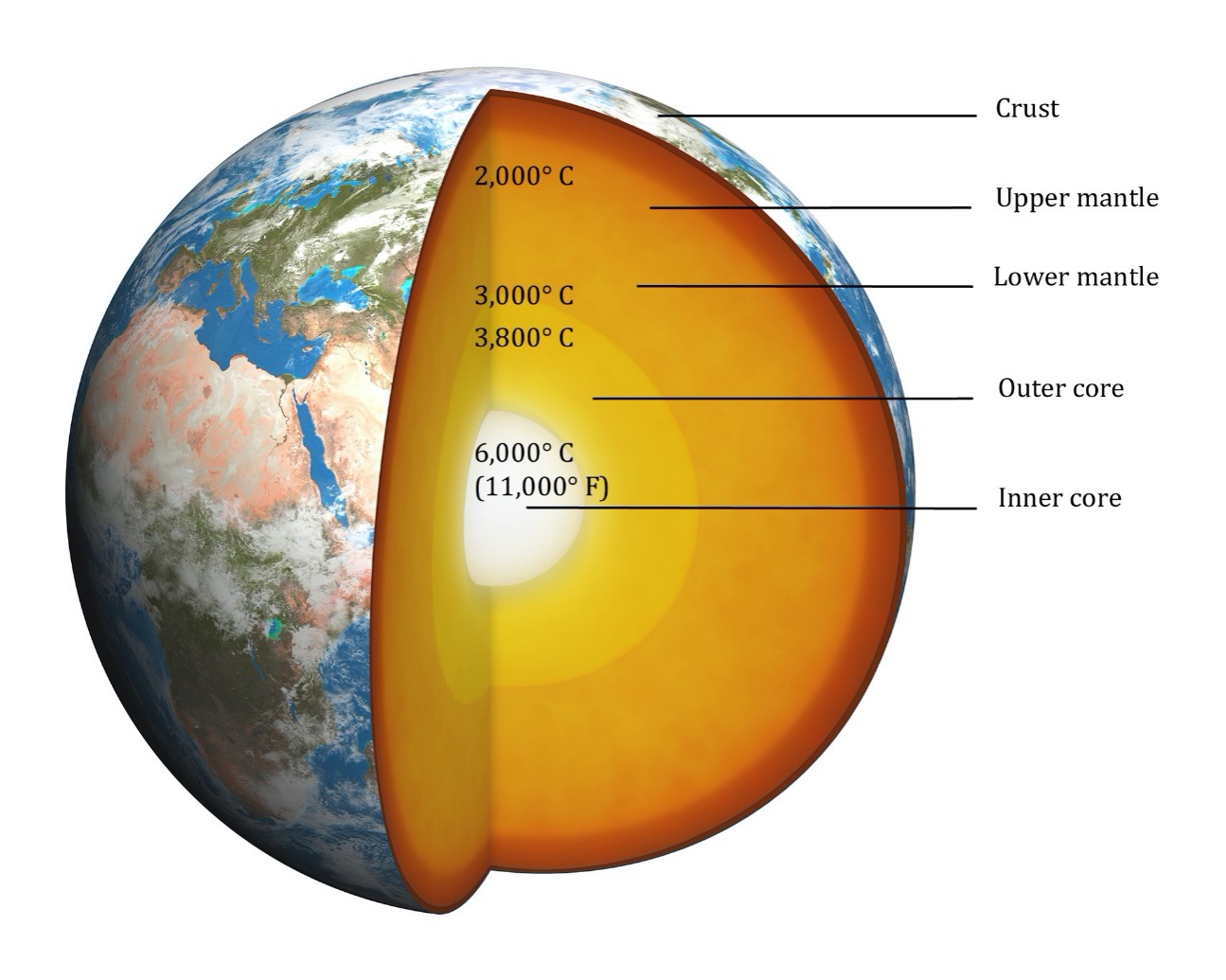
Earth core

  • 99% of the earth is > 1000 \(^o\)C
  • Heated by radioactive decay
  • P up to 4 Mbar
  • 0.07, 0.1 W/m\(^2\) (land, ocean)
    • 9.2 CMO/yr
  • total heat energy 1.26E31 J
    • 5.4E27 J in the crust
  • Thermal gradient ~25 \(^o\)C/km (100 \(^o\)C at 4 km).

Geothermal plants

 

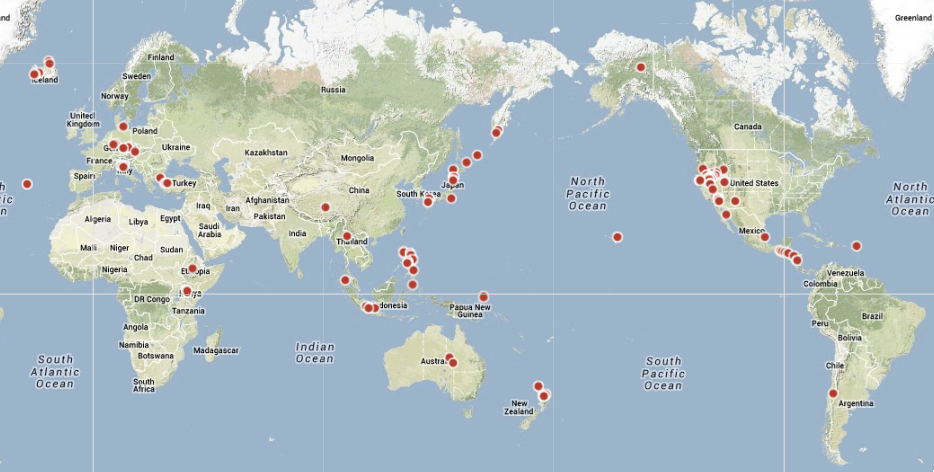
  • The the prevalence of plants around the “ring of fire”

US Geothermal potential

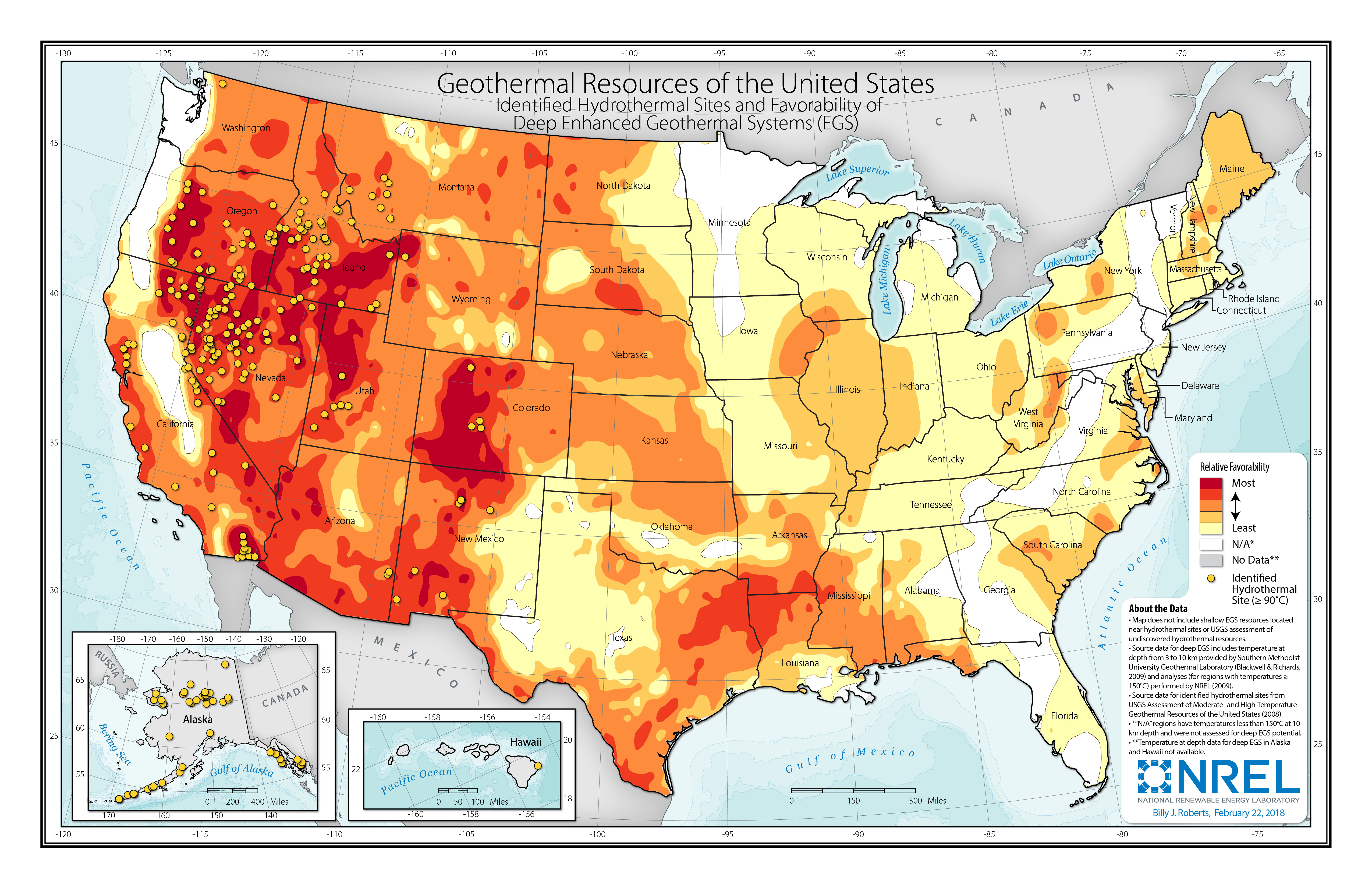
Geothermal production (direct heat)

Lund and Boyd, Direct Utilization of Geothermal Energy 2015 Worldwide Review, World Geothermal Congress, 2015

Geothermal heat, power

  • Two applications
    • Direct heat
    • Electricity generation
      • First geothermal plant: Tuscany Italy 1904
  • Average size > 10 MW = 153 MW
  • Types: dry, flash (most common), binary

Geysers Geothermal Complex

Hot dry rock (HDR)

  • Summary of papers and articles
  • Enhanced geothermal system (EGS)
  • No natural water table
  • Rock fracturing
  • Two wells drilled: injection and production
  • Depth issues

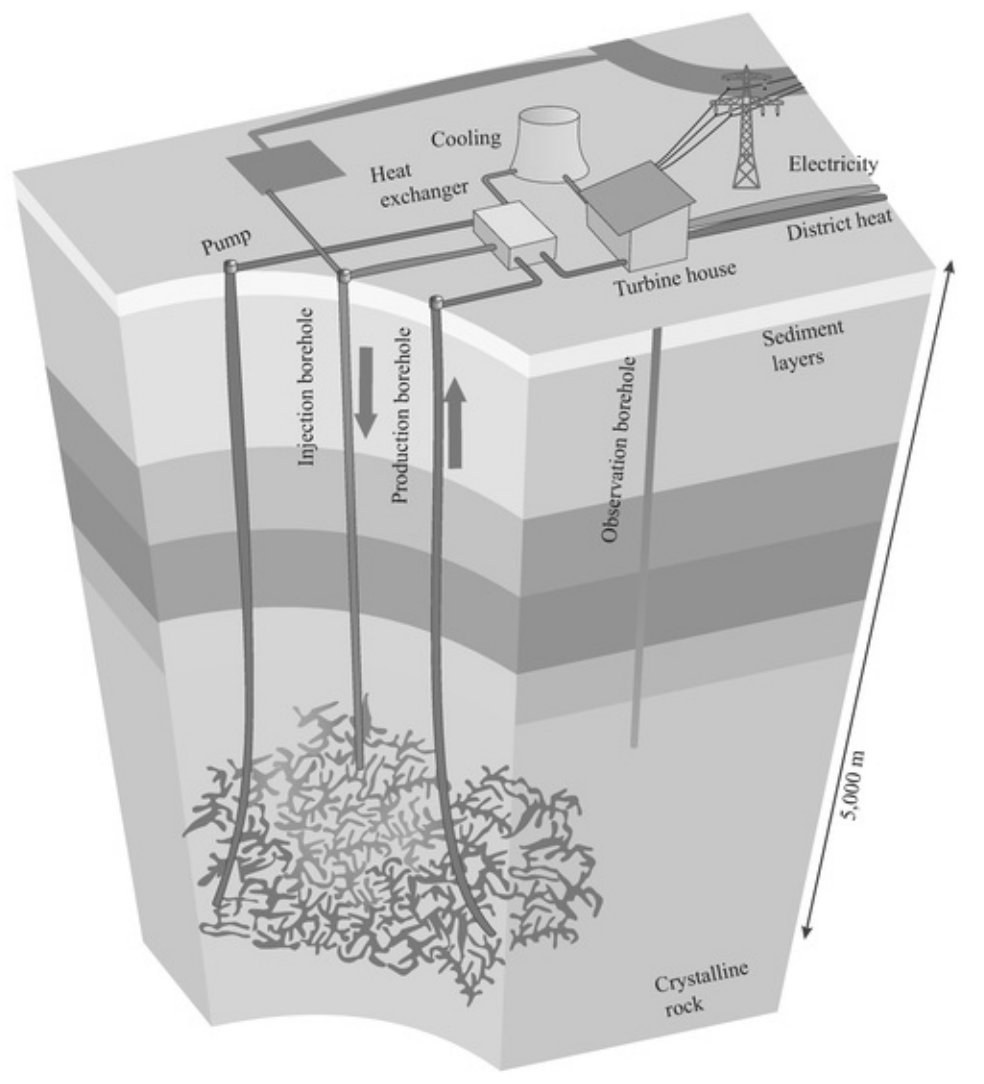
Issues

  • Renewable
  • Environmentally benign (other than land use)
  • Lower temperatures use the organic Rankine cycle (ORC)
  • Low efficiencies at lower temperatures \(\rightarrow\) direct heat is more cost effective
  • Drilling costs
  • Resource depletion (run out of steam \(\rightarrow\) inject water, e.g., Geysers Complex)

Article